Differentiation
Differentiation Rules
Note:
Chain Rule
For 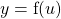 and
and 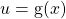 ,
,
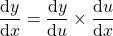
Further Differentiation Rules (Chain Rule)
Product Rule
Quotient Rule
Gradient Of Curve
From 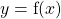 ,
,
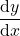 is the gradient of the curve.
is the gradient of the curve.
Gradient Of Tangent & Normal
From 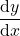 , substitute
, substitute 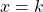 to get
to get 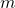 (gradient of tangent).
(gradient of tangent).
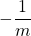 is the gradient of the normal.
is the gradient of the normal.
Increasing & Decreasing Functions
1. For increasing functions, 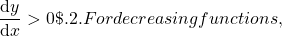 \dfrac{\text{d}y}{\text{d}x}<0
\dfrac{\text{d}y}{\text{d}x}<0![Rendered by QuickLaTeX.com .[/stextbox] [stextbox caption="Rates Of Change" stextbox id="info"]](https://teach.sg/wp-content/ql-cache/quicklatex.com-d6569e51e60b9302b073cb6a581e8ede_l3.png) \dfrac{\text{d}y}{\text{d}t}=\dfrac{\text{d}y}{\text{d}x}\times\dfrac{\text{d}x}{\text{d}t}
\dfrac{\text{d}y}{\text{d}t}=\dfrac{\text{d}y}{\text{d}x}\times\dfrac{\text{d}x}{\text{d}t}![Rendered by QuickLaTeX.com [/stextbox] [stextbox caption="First Derivative Test" stextbox id="default"]If](https://teach.sg/wp-content/ql-cache/quicklatex.com-db52040a4d707e532107dfe5b3a967db_l3.png) \dfrac{\text{d}y}{\text{d}x}=0
\dfrac{\text{d}y}{\text{d}x}=0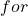 x=k
x=k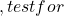 k^-
k^- k
k k^+
k^+
![Rendered by QuickLaTeX.com . Maximum point: <span class="ql-right-eqno"> </span><span class="ql-left-eqno"> </span><img src="https://teach.sg/wp-content/ql-cache/quicklatex.com-9f9378ea29ee6f36752bf951c2f47e82_l3.png" height="61" width="142" class="ql-img-displayed-equation quicklatex-auto-format" alt="\[\begin{array}{|c|c|c|c|} \hline x & k^- & k & k^+\\\hline \dfrac{\text{d}y}{\text{d}x} & + & 0 & - \\ \hline \end{array}\]" title="Rendered by QuickLaTeX.com"/> Minimum point: <span class="ql-right-eqno"> </span><span class="ql-left-eqno"> </span><img src="https://teach.sg/wp-content/ql-cache/quicklatex.com-e34eb65dd85078db31a0864a230b4e33_l3.png" height="61" width="142" class="ql-img-displayed-equation quicklatex-auto-format" alt="\[\begin{array}{|c|c|c|c|} \hline x & k^- & k & k^+\\\hline \dfrac{\text{d}y}{\text{d}x} & - & 0 & + \\ \hline \end{array}\]" title="Rendered by QuickLaTeX.com"/> Inflexion point: <span class="ql-right-eqno"> </span><span class="ql-left-eqno"> </span><img src="https://teach.sg/wp-content/ql-cache/quicklatex.com-6c08639adf6fd54c2707aa0c3de17366_l3.png" height="98" width="142" class="ql-img-displayed-equation quicklatex-auto-format" alt="\[\begin{array}{|c|c|c|c|} \hline x & k^- & k & k^+\\\hline \dfrac{\text{d}y}{\text{d}x} & + & 0 & + \\\hline \dfrac{\text{d}y}{\text{d}x} & - & 0 & - \\\hline \end{array}\]" title="Rendered by QuickLaTeX.com"/>[/stextbox] [stextbox caption="Second Derivative Test" stextbox id="download"]1. If](https://teach.sg/wp-content/ql-cache/quicklatex.com-e32cc11ea31a8c16a576e42aa4bd37f5_l3.png)
\dfrac{\text{d}^2y}{\text{d}x^2}<0\$, it is a maximum point.
2. If ![]() , it is a minimum point.
, it is a minimum point.
3. If ![]() , need to do first derivative test.
, need to do first derivative test.
