Proofs In Plane Geometry
Properties Of Circles
1. tan.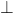 
rad.
2. rt.
in semicircle
3.s in same seg.
4. at centre = 2 at circ.
5.s in opp. seg. (
)
6. tan. from ext. pt.
rad.2. rt.
in semicircle3.
s in same seg.4.
at centre = 2 at circ.5.
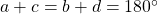s in opp. seg. ()6. tan. from ext. pt.
Congruent & Similar Triangles
![\[\begin{array}{|c|c|} \hline \text{Congruent triangles} & \text{Similar triangles}\\\hline \text{SSS, SAS, AAS, RHS} & \text{SSS, SAS, AAA}\\\hline \end{array}\]](https://teach.sg/wp-content/ql-cache/quicklatex.com-8b94e533fc9065620aa9998914687f51_l3.png "Rendered by QuickLaTeX.com")
Midpoint Theorem
If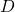 
and 
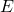are the midpoints of 
and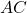 
respectively, then 
// 
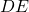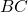and 
.
and are the midpoints of and respectively, then // and .Tangent-Chord Theorem (Alternate Segment Theorem)
If 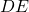is a tangent to the circle at 
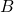, then 
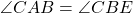and 
.
is a tangent to the circle at , then and 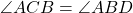.