Trigonometric Functions, Identities & Equations

Special Angles

$\begin{array}{|c|c|c|c|c|c|} \hline \theta & 0^{\circ}& 30^{\circ} & 45^{\circ} & 60^{\circ} & 90^{\circ} \\ \hline \sin\theta & \frac{\sqrt{0}}{2}=0 & \frac{\sqrt{1}}{2}=\frac{1}{2} & \frac{\sqrt{2}}{2} & \frac{\sqrt{3}}{2} & \frac{\sqrt{4}}{2}=1 \\ \hline \cos\theta & \frac{\sqrt{4}}{2}=1 & \frac{\sqrt{3}}{2} & \frac{\sqrt{2}}{2} & \frac{\sqrt{1}}{2}=\frac{1}{2} & \frac{\sqrt{0}}{2}=0 \\ \hline \tan\theta & 0 & \frac{1}{\sqrt{3}} & 1 & \sqrt{3} & - \\ \hline \end{array}$

Reciprocal Functions

$1.\,\text{cosec }\theta=\dfrac{1}{\sin\theta}\$$ 2.\,\sec\theta=\dfrac{1}{\cos\theta}\$
$3.\,\cot\theta=\dfrac{1}{\tan\theta}$

Negative Functions

$1.\,\sin(-\theta)=-\sin\theta$
$2.\,\cos(-\theta)=\cos\theta$
$3.\,\tan(-\theta)=-\tan\theta$

Tangent & Cotangent

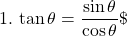
$1.\,\tan\theta=\dfrac{\sin\theta}{\cos\theta}\$$ 2.\,\cot\theta=\dfrac{\cos\theta}{\sin\theta} $[/stextbox] [stextbox caption="Trigonometric Identities" stextbox id="download"]$ 1.\,\sin^2{\theta} + \cos^2{\theta} = 12.\,\sec^2{\theta}=1+\tan^2{\theta}3.\,\text{cosec}^2{\theta}=1 + \cot^2{\theta} $[/stextbox] [stextbox caption="Addition Formulae" stextbox id="info"]$ 1.\,\sin(A \pm B) = \sin A\cos B \pm \cos A \sin B2.\,\cos(A \pm B) = \cos A \cos B \mp \sin A \sin B3.\,\tan(A \pm B) = \dfrac{\tan A \pm \tan B}{1 \mp \tan A \tan B} $[/stextbox] [stextbox caption="Double Angle Formulae" stextbox id="default"]$ 1.\,\sin 2A = 2\sin A \cos A2.\,\cos2A = \cos^2A – \sin^2A= 2 \cos^2A -1= 1 – 2\sin^2A3.\,\tan 2A = \dfrac{2\tan A}{1-\tan^2A} $[/stextbox] [stextbox caption="R-Formulae" stextbox id="download"]For$ a > 0, b > 0, 0^\circ < \alpha < 90^\circ $,$ 1.\,a\sin \theta \pm b \cos \theta = R \sin (\theta \pm \alpha)2.\,a \cos \theta \pm b \sin \theta = R \cos (\theta \mp \alpha) $where$ R=\sqrt{a^2+b^2}, \tan\alpha=\dfrac{b}{a}$.